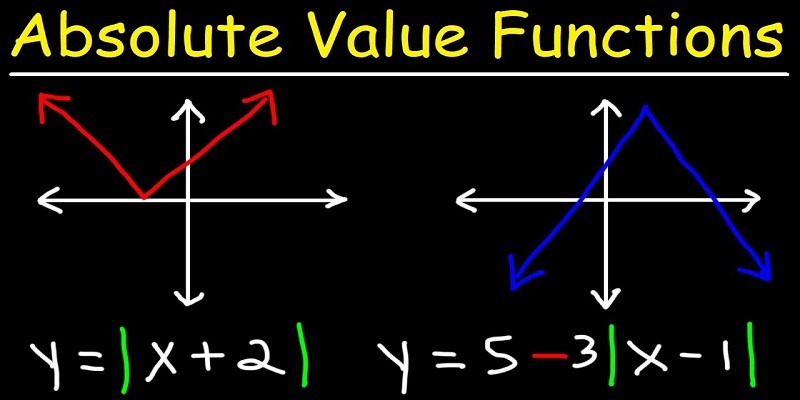
The range of the parent absolute value function is all real numbers. The parent absolute value function has a range that extends from negative infinity to positive infinity, covering all possible values of y.
It is a V-shaped graph that is symmetric about the y-axis, with the vertex at the origin. In other words, the range of the parent absolute value function includes all positive and negative numbers, including zero. The graph of the parent absolute value function is always above or on the x-axis and is never below it.
This allows the function to cover the entire range of real numbers without any gaps or exclusions.
What Is The Parent Absolute Value Function?
The parent absolute value function is a linear function that outputs the distance of a number from zero. The range of the parent absolute value function is always greater than or equal to zero.
Definition And Formula Of The Parent Absolute Value Function:
The parent absolute value function is a fundamental mathematical function with a distinct “V” shape. It describes the distance of a number from zero, regardless of whether it is positive or negative. The formula for the parent absolute value function is written as:
|x| = x if x is greater than or equal to 0,
| x | = -x if x is less than 0.
Here, the vertical bars, also known as absolute value bars, are used to represent the function. Let’s explore the basic shape and characteristics of this function.
The Basic Shape And Characteristics Of The Parent Function:
- The parent absolute value function consists of two linear segments intersecting at the origin (0, 0) on a coordinate plane.
- It has a V-shaped graph, symmetric with respect to the y-axis.
- The left segment of the V has a positive slope of 1, while the right segment has a negative slope of -1.
- The function’s value is always non-negative, resulting in a graph that lies above or touches the x-axis.
- The vertex of the V-shape is the point where the two linear segments meet, at (0, 0).
- As x approaches positive or negative infinity, the function also approaches positive infinity, indicating an unbounded range in both directions.
- The domain of the parent absolute value function is all real numbers, while the range is the set of non-negative real numbers.
To summarize, the parent absolute value function is represented by a V-shaped graph with a vertex at the origin. It measures the distance between a number and zero, disregarding its sign. This function offers useful insights into various mathematical concepts and serves as the basis for understanding transformations and variations of the absolute value function.
The Range Of The Parent Absolute Value Function
Understanding the range of the parent absolute value function is vital for determining the domain of the function. By analyzing the behavior of the function, we can identify the set of all possible output values, providing valuable insights into its overall characteristics.
The range of a function refers to the set of all possible output values it can produce. When it comes to the parent absolute value function, there are certain characteristics that define its range. Let’s explore the concept of range in mathematics and how it applies to the parent absolute value function.
Explaining The Concept Of Range In Mathematics
Understanding the concept of range in mathematics is essential to grasp the behavior of functions. Here are some key points to consider:
- Range refers to the set of all possible y-values (outputs) for a given function.
- The range can be finite or infinite, depending on the function.
- In the case of the parent absolute value function, the range consists of all non-negative real numbers (including zero).
Determining The Range Of The Parent Absolute Value Function Algebraically
To determine the range of the parent absolute value function algebraically, we need to analyze its equation and properties. Here’s what you need to know:
- The equation for the parent absolute value function is y = |x|.
- Since the absolute value function always returns a non-negative value, the range of the parent absolute value function is [0, +∞).
Graphical Representation Of The Range Of The Parent Function
Graphically representing the range of the parent absolute value function can provide a visual understanding of its behavior. Consider the following:
- When graphed, the parent absolute value function forms a “V” shape, centered at the origin.
- The lowest point of the graph occurs at the vertex (0,0), representing the minimum value of 0.
- As the function extends towards both positive and negative x-values, the y-values increase indefinitely.
- Consequently, the graph extends upwards along the y-axis infinitely, displaying the open range from 0 to positive infinity.
Understanding the range of the parent absolute value function is crucial in comprehending its behavior. Algebraically, the range consists of all non-negative real numbers, while graphically, the function extends indefinitely along the positive y-axis. By grasping these concepts, you can confidently work with the parent absolute value function and analyze its properties.
Describing The Range Of The Parent Absolute Value Function
The parent absolute value function has a range that includes all real numbers greater than or equal to zero. It represents the distance from zero on the number line.
The parent absolute value function, also known as |x|, is a fundamental mathematical concept. Understanding its range is key to grasping its behavior. In this section, we will delve into three crucial factors that influence the range of the parent absolute value function: the horizontal shift, the vertical shift, and the amplitude.
Analyzing The Horizontal Shift And Its Effect On The Range:
When the parent absolute value function is horizontally shifted, it affects the range in the following ways:
- If the horizontal shift is positive, the range will start at a higher value than the parent function.
- If the horizontal shift is negative, the range will start at a lower value than the parent function.
- The horizontal shift does not impact the overall width or symmetry of the range.
Exploring The Vertical Shift And Its Impact On The Range:
The vertical shift of the parent absolute value function influences the range as follows:
- If the vertical shift is positive, the range will move upward, resulting in higher values overall.
- If the vertical shift is negative, the range will shift downward, resulting in lower values overall.
- The vertical shift does not affect the symmetry or width of the range.
Considering The Role Of The Amplitude In Determining The Range:
The amplitude is a vital factor in defining the range of the parent absolute value function. Its impact can be summarized as follows:
- The amplitude determines the vertical stretch or compression of the function.
- When the amplitude is greater than 1, the range extends higher and lower than the parent function.
- When the amplitude is between 0 and 1, the range becomes narrower, with values concentrated closer to the x-axis.
- The amplitude does not affect the horizontal position or symmetry of the range.
Understanding the various influences on the range of the parent absolute value function is crucial for interpreting and analyzing its graph. By considering the horizontal shift, vertical shift, and amplitude, we can gain valuable insights into the behavior and limitations of this essential mathematical concept.

Credit: www.screendaily.com
Frequently Asked Questions For Which Describes The Range Of The Parent Absolute Value Function?
What Is The Parent Absolute Value Function?
The parent absolute value function is a linear function that represents the absolute value of a number.
How Does The Parent Absolute Value Function Look Like?
The parent absolute value function is represented by the formula f(x) = |x|, where |x| denotes the absolute value of x.
What Is The Range Of The Parent Absolute Value Function?
The range of the parent absolute value function is all real numbers greater than or equal to zero.
What Shapes Does The Parent Absolute Value Function Create?
The parent absolute value function creates a V-shaped graph, also known as a “V-curve” or a “V-graph”.
Conclusion
The parent absolute value function is a fundamental concept in mathematics that describes the range of values it can produce. Through its characteristic v-shape, the function extends from negative infinity to positive infinity, encompassing all real numbers. By understanding the range of the parent absolute value function, we gain insight into various related functions that involve changes such as translations, reflections, and stretches.
This knowledge allows us to solve equations and inequalities involving absolute values with ease, making it a crucial skill in algebraic problem-solving. Furthermore, comprehending the range of the parent absolute value function aids in grasping real-world applications. From graphing temperature fluctuations to tracking the movements of objects, absolute values find their way into various fields.
By applying this understanding, we can interpret data accurately and make informed decisions. Mastering the range of the parent absolute value function equips us with a valuable tool that enhances our mathematical proficiency and problem-solving abilities. So, take the time to study and practice this concept, as it will undoubtedly open doors to countless mathematical possibilities.