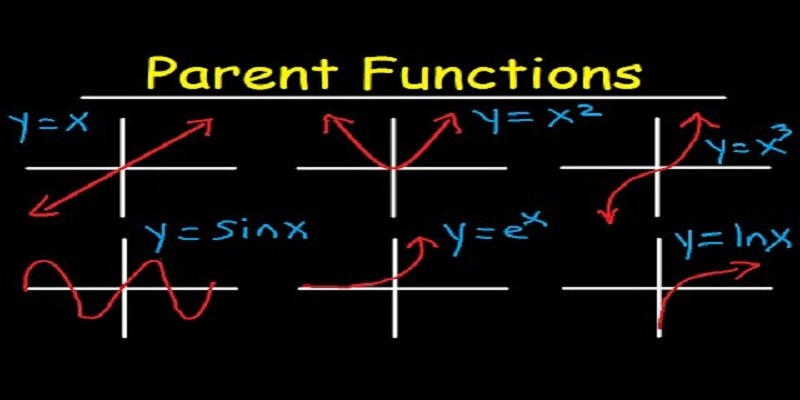
A parent function is the most basic form of a mathematical function. It serves as a template for producing other related functions with different transformations and variations.
By analyzing the properties of the parent function, we can gain a better understanding of its corresponding family of functions. This knowledge allows us to recognize patterns, make predictions, and solve problems in mathematics. Parent functions commonly encountered include linear, quadratic, cubic, exponential, logarithmic, and trigonometric functions.
Each parent function has distinct characteristics and features that define its behavior. Understanding parent functions forms the foundation for studying and manipulating more complex functions in algebra and calculus. Mastering the concept of a parent function is crucial for comprehending the principles and applications of algebraic and mathematical equations.
What Are Parent Functions?
A parent function is a basic function that serves as a starting point for creating other functions. It provides a framework for understanding and graphing different types of functions.
Definition Of Parent Functions
A parent function is a basic function that serves as a template or blueprint for a family of functions. It acts as a starting point from which other functions can be derived or transformed. In other words, parent functions exhibit the fundamental characteristics and behavior of a particular type of function.
These functions play a vital role in mathematics by providing a foundation for understanding and analyzing various types of functions.
Importance Of Parent Functions In Mathematics
Understanding parent functions is crucial in mathematics for several reasons:
- Building Block: Parent functions are like building blocks that help mathematicians construct and understand more complex functions. By studying the properties and behavior of parent functions, we can easily identify similar patterns in other functions belonging to the same family.
- Function Transformation: Parent functions serve as a reference point when transforming functions. By manipulating the parent function through translations, reflections, stretches, and compressions, we can create a wide variety of functions that possess similar properties.
- Graphical Analysis: Parent functions help us analyze the graphical representations of functions. By studying the key features and shape of the parent function, we can make predictions about the behavior, domain, range, symmetry, and intercepts of other functions.
- Function Classification: Parent functions aid in classifying different types of functions. By recognizing the characteristics of a particular parent function, we can determine its category, such as linear, quadratic, exponential, or trigonometric.
- Problem Solving: Parent functions provide a framework for solving mathematical problems. By utilizing the known properties and behavior of parent functions, we can apply them to real-world situations and make accurate predictions or calculations.
Understanding the concept of parent functions is essential for comprehending and navigating the vast world of mathematical functions. With a solid understanding of these foundational functions, mathematicians can explore and apply more complex functions with confidence and precision.
Credit: www.youtube.com
Common Types Of Parent Functions
A parent function refers to the common types of functions that form the foundation for other functions. These functions include linear, quadratic, cubic, absolute value, square root, and reciprocal functions. Understanding parent functions is essential in mathematics as they serve as a starting point for graphing and analyzing more complex functions.
The concept of parent functions is fundamental to understanding the behavior of mathematical functions. These functions serve as the building blocks from which more complex functions are derived. In this section, we will explore some of the most common types of parent functions and their characteristics.
Linear Parent Function
- A linear parent function is a basic function that represents a straight line on a coordinate plane.
- It is defined by the equation y = mx + b, where m represents the slope of the line and b represents the y-intercept.
- The slope determines the steepness of the line, while the y-intercept represents the point at which the line crosses the y-axis.
Quadratic Parent Function
- A quadratic parent function is a function that represents a parabolic curve.
- It is defined by the equation y = ax^2 + bx + c, where a, b, and c are constants.
- The leading coefficient (a) determines the direction and shape of the parabola. A positive value for a creates a U-shaped parabola, while a negative value creates an inverted U shape.
Cubic Parent Function
- A cubic parent function is a function that represents a curve with an S-shaped pattern.
- It is defined by the equation y = ax^3 + bx^2 + cx + d, where a, b, c, and d are constants.
- The degree of the polynomial determines the number of bends in the curve. A cubic function has two bends, which contribute to its distinctive shape.
Absolute Value Parent Function
- An absolute value parent function is a function that represents a V-shaped graph.
- It is defined by the equation y = |x|, where |x| denotes the absolute value of x.
- The graph of this function is symmetric with respect to the y-axis.
Square Root Parent Function
- A square root parent function is a function that represents the principal square root of a number.
- It is defined by the equation y = √x, where √x denotes the square root of x.
- The graph of this function is a curve that starts at the origin (0,0) and extends to the right.
By understanding these common types of parent functions, you will have a solid foundation for exploring more complex mathematical concepts. Each parent function has its own unique characteristics, allowing mathematicians and scientists to describe and analyze various phenomena using these fundamental building blocks.
Characteristics Of Parent Functions
A parent function is the simplest form of a mathematical function. It serves as a template for related functions, and understanding its characteristics is crucial for analyzing and graphing various mathematical equations.
What Is A Parent Function?
If you’re exploring the world of functions and graphing, understanding parent functions is an essential building block. Parent functions serve as the foundation for various types of functions and provide a reference point for understanding their characteristics. In this section, we will delve into the characteristics of parent functions, including graphing, domain and range, transformations, symmetry, and end behavior.
Graphing Parent Functions:
- Parent functions are the basic forms of functions that can be modified to create other functions.
- Graphing parent functions allows us to visualize the shape and behavior of these functions.
- The graph of a parent function can typically be recognized by its distinctive shape and general trend.
Domain And Range Of Parent Functions:
- The domain of a parent function refers to the set of all possible input values for the function.
- The range of a parent function represents the set of all possible output values for the function.
- The domain and range of a parent function can help us identify the boundaries within which the function operates.
Transformations Of Parent Functions:
- Transformations involve modifying the parent function by changing its shape, position, or scale.
- Common transformations include vertical and horizontal shifts, reflections, and stretches or compressions.
- By applying transformations, we can create new functions that retain certain characteristics of the parent function while introducing changes.
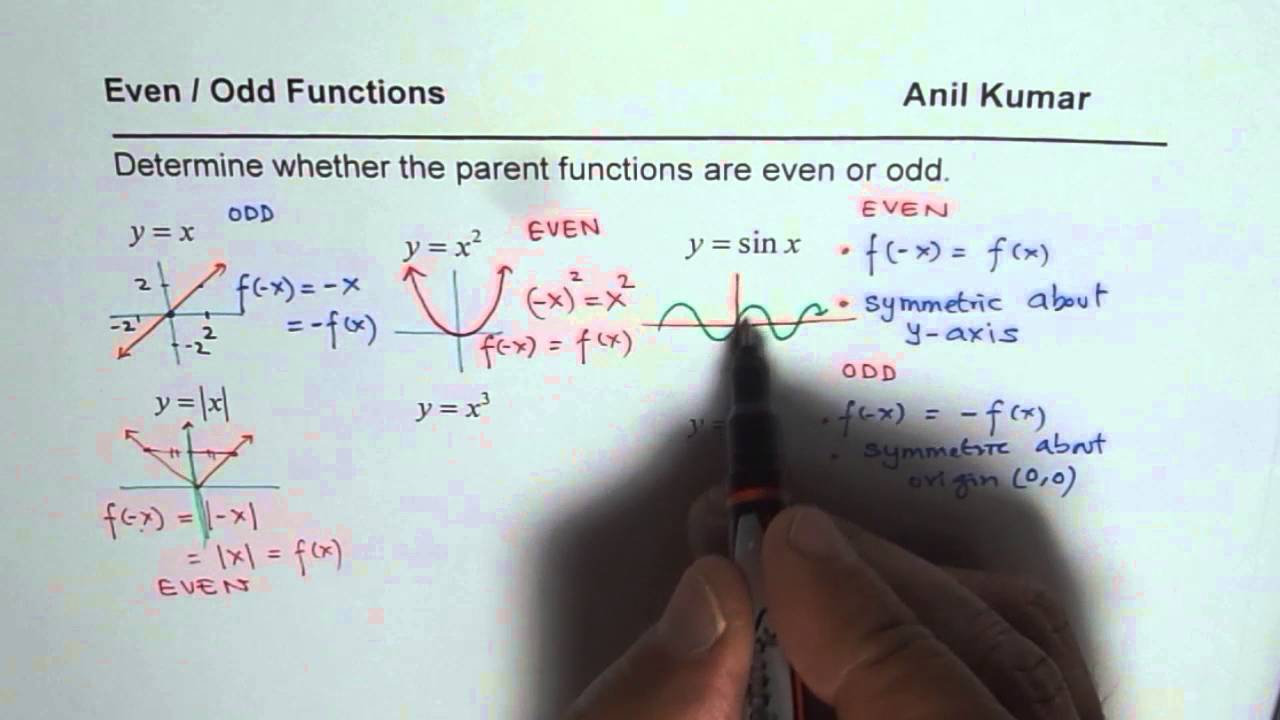
Symmetry And End Behavior Of Parent Functions:
- Symmetry refers to the property of a graph that remains unchanged when reflected across a certain line.
- Parent functions can exhibit different types of symmetry, such as even symmetry (y-axis symmetry) or odd symmetry (origin symmetry).
- End behavior refers to the behavior of the graph as it approaches positive or negative infinity.
- Understanding the symmetry and end behavior of parent functions provides insights into their overall shape and characteristics.
By gaining a solid understanding of these characteristics, you’ll be better equipped to analyze and work with functions in various mathematical contexts. Now that we have explored the characteristics of parent functions, let’s move on to exploring specific types of parent functions and their unique properties.
Frequently Asked Questions Of What Is A Parent Function?
What Is A Parent Function In Math?
A parent function is a basic function that serves as a template for other functions.
What Are The 4 Parent Functions?
The 4 parent functions are linear, quadratic, cubic, and square root.
What Is Parent Functions And Transformations?
Parent functions are basic functions that act as a starting point for transformations in mathematics.
What Is The Difference Between A Parent Function And A Family Function?
A parent function is the most basic form of a function, while a family function is a group of functions related to each other through a common equation.
What Is A Parent Function?
A parent function is the simplest form of a mathematical function that serves as a template for other related functions.
Conclusion
Understanding parent functions is crucial in mathematics as they serve as the building blocks for various functions. By knowing the characteristics and transformations of parent functions, we can easily identify and analyze more complex functions. The parent functions—linear, quadratic, cubic, square root, absolute value, and reciprocal functions—are essential tools for modeling and interpreting real-world situations.
Additionally, they help in graph plotting and identifying key features such as intercepts, maximums, minimums, and symmetry. Moreover, knowing the parent functions enables us to comprehend advanced concepts like transformations, stretches, translations, and reflections. Applying these transformations to parent functions allows us to create customized functions that accurately represent data and solve specific problems.
Consequently, having a solid understanding of parent functions empowers us to navigate through complex mathematical concepts with ease, making them an essential aspect of mathematical education. By grasping parent functions, we lay a strong foundation for further mathematical exploration and problem-solving.